Page 53 - Msingi Afrika Magazine Issue 11
P. 53
MY AFRIKA
4+4...". So in the demonstration to get 2,4,6,8,10 in our second So we write down our resulting
of the proof of the Ishango row as above (but starting from sequence of primes in the series
sieve to follow, it will be seen 3 on the bone itself to double to 1-10 as displayed on the bone.
that this conception of copying 6). 5
indicated above exists in societ- 7
ies of the region and suffices as For completeness we include
a means of doubling numbers. all doubling from 1 upwards In the next step the elimina-
Furthermore, identifying num- although this begins at 3. The tion process described above
bers that are composed of "cop- third row shows the numbers is repeated to obtain the re-
ies" of other numbers suffices as produced when a number and maining primes 11,13,17,19 in
a means of identifying composite its double are added, so that for column G, when eliminating
or non-prime numbers, as we instance, 9 is obtained from the non-primes from the sequence
shall see shortly. sum of 3 and 6. We note that like 11,12,13,14,15,16,17,18,19,20. As
all even numbers, 6 is composed before, we begin by doubling; but
Lastly, it is curious that the of 2 "copies" of another num- this time we double the numbers
Ishango mathematicians do not ber, 6 is composed of copies of from 6 to 10 as below and pro-
exhibit the numbers 1 and 2 even 3 (that is 6 = 3+3 ).We note all ceed exactly as before.
though these numbers are patent- numbers beyond 6 in the 3rd row
ly employed in their calculations. are composed of copies of other
Is it possible that these ancient numbers.
mathematicians had a sacred
reverence for these numbers In our next step all numbers that
which has partially hidden their are composed of copies of oth-
ingenious sieve method for de- ers are eliminated from the series
termining the small primes? For 1,2,3,4,5,6,7,8,9,10. The elimi- Figure 4. The Doubling of Numbers 6 to 10.
instance, the number two is used nated numbers are shown with a
in doubling; yet nowhere is two strike as below. So doubling 6 to 10 we get in the
displayed on the bone. Also the second row, 12,14,16,18,20. As
number 1, predecessor of 2, is before, the third row shows the
not displayed overtly. Alternative- numbers produced by a number
ly, it may simply be the case that and its double added, so that for
as a way of shortening the length Figure 3. The Elimination of Numbers instance, 24 is obtained from the
of calculations they have opted Composed of "Copies" of Others sum of 8 and 16. By inspection
to omit the numbers and save We note above that all of the of the composition of numbers
time, effort and resources. even numbers are composed of previously generated by our
two copies of another number doubling process it is plain to see
5. Proof of the Ishango Math- and so are eliminated. Also 9 = that all of the even numbers are
ematical Sieve 3+6 from our calculations on copies of others, as before. Fur-
column M. We also know that thermore, we also note that one
Our ancient Ishango mathemati- 6 is 2 copies of 3 and 6 = 3+3. odd number in the range 10-20 is
cians would have begun the siev- Hence 9 = 3 + 6 = 3 + 3 + 3. also composed of copies of oth-
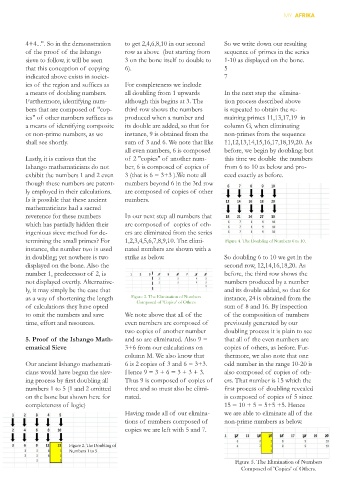
ing process by first doubling all Thus 9 is composed of copies of ers. That number is 15 which the
numbers 1 to 5 (1 and 2 omitted three and so must also be elimi- first process of doubling revealed
on the bone but shown here for nated. is composed of copies of 5 since
completeness of logic) 15 = 10 + 5 = 5+5 +5. Hence
Having made all of our elimina- we are able to eliminate all of the
tions of numbers composed of non-prime numbers as below.
copies we are left with 5 and 7.
Figure 2. The Doubling of
Numbers 1 to 5
WWW.MSINGIAFRIKAMAGAZINE.COM ISSUE 11 | MAR/APRIL 2021 53
Figure 5. The Elimination of Numbers
Composed of "Copies" of Others.